Speed and Velocity
Overview
Speed and velocity are both terms that we have come to associate with motion of some kind. In order to define these terms, however, we need some point of reference. An object surrounded by empty space in all directions cannot be said to be moving, let alone moving at a particular speed or velocity, for the simple reason that we have no point of reference against which these things can be measured.
In this page we will examine the concepts of speed and velocity and attempt to provide some definitions and a simple "frame of reference" within which those definitions can have a meaningful context. The reader should note that, despite the fact that we are primarily approaching this topic in the context of classical mechanics we will, of necessity, be touching on issues related to special relativity.
Notational conventions
The symbols used to represent quantities such as speed, velocity, distance and displacement vary from one source to the next. In these pages, we will be adopting the convention that symbols representing scalar quantities (like speed and distance) will appear in a normal typeface, while vector quantities (like velocity and displacement) will appear in a bold typeface. A brief summary of the symbols used in this page is given below.
- v - speed, in metres per second (m/s or m s-1)
- v - velocity, in metres per second (m/s or m s-1)
- s - distance, in metres (m)
- x - displacement, in metres (m), for one-dimensional motion
- s - displacement, in metres (m), for two- or three-dimensional motion
- t - time, in seconds (s)
Speed
Informally, we can think of speed as the rate at which an object covers a given distance - a concept first expressed by Galileo. An object moving at high speed will cover a specified distance in less time than an object that is moving more slowly. We could express this a little differently by saying that, in a given period of time, a fast-moving object will cover a greater distance than a slow-moving object. It may seem to you that we are stating the obvious here, but we have to start the discussion somewhere.
Interestingly enough, speed is not one of the base quantities defined in the International System of Units. Rather, it is one of the derived SI quantities, and is defined as the quotient of two base units - length (or distance) in metres and time in seconds. Speed is thus expressed in units of metres per second (m/s or m s-1). We can express this more formally as:
| speed (m/s) = | distance (m) |
| time (s) |
You will no doubt be familiar with somewhat different units of speed, depending on where you live. In the USA or the UK, for example, we often talk about speed in terms of miles per hour to express how fast a vehicle is travelling. In mainland Europe, it would be kilometres per hour. In terms of describing the speed of things we encounter on a daily basis these units are simply easier to visualise. Physicists, however, need to be a little more precise.
Speed is a scalar (i.e. one-dimensional) quantity - it has magnitude, but not direction. When we talk about speed on a daily basis, we use a number to describe it. We might say, for example, that we are driving along the road at forty-five miles per hour. We rarely talk about the direction in which we are travelling (most of us are probably unaware of our precise direction at any given time anyway). Physicists, on the other hand, must often also concern themselves with the direction in which an object is moving, as well as its speed. This means they need to know its velocity.
Velocity
Velocity is a vector quantity. It has a magnitude that describes the rate at which an object is moving (i.e. its speed), and it specifies the direction in which the object is moving. Speed is thus a component of velocity. The velocity of an object is defined as the rate of change in its position with respect to a specific reference point, or within some defined frame of reference.
When talking about everyday things, we sometimes use the word velocity instead of speed, but this is an incorrect use of the word. Like speed, velocity is a function of time, but it also specifies the direction of motion. If we say we are driving at sixty miles per hour, we are describing our speed. If, on the other hand, we say we are driving North at sixty miles an hour, we are describing our velocity - albeit somewhat informally.
Velocity is commonly indicated in equations using the lower-case letter v, which is sometimes also used for speed (remember, though, that symbols representing vector quantities are usually printed in bold typeface to distinguish them from symbols that represent scalar quantities). Like speed, the SI unit of velocity is metres per second (m/s or m s-1). The basic formula for velocity is therefore very similar to that for speed:
| velocity (m/s) = | displacement (m) |
| time (s) |
Note that we use the word displacement rather than distance. This is because, whereas the speed of an object depends on the total distance travelled by the object (irrespective of direction) and the time taken to travel that distance, velocity is dependent only on the shortest distance between the start and end points.
This essentially means that if an object's movement takes it back to the point from which it started, its displacement - and therefore its net velocity for that movement - will be zero, regardless of the total distance travelled or the speed at which it has moved. Before we talk further about speed or velocity, therefore, we will look a little more closely at the concept of displacement.
Displacement
Displacement is a vector quantity and is defined as the shortest distance between the initial position and the final position of an object. In the diagram below, you can see that the path travelled between the point of origin and the endpoint (represented by the solid line) changes direction several times. The displacement vector, represented by the dotted line, is the shortest path between these two points. It has both length and direction.
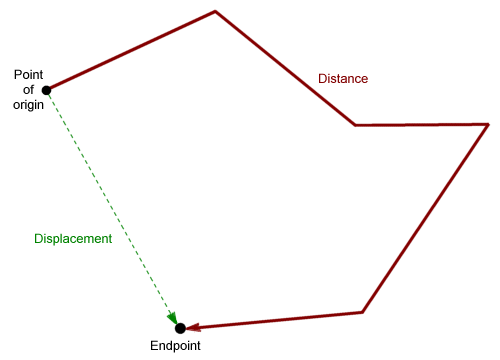
Total displacement is represented as the shortest path between the point of origin and the endpoint
Note that each leg of the path shown in the diagram above is a displacement vector in its own right. The displacement vector for the complete path travelled is the sum of the individual displacement vectors. Note also that, assuming the point of origin and the endpoint are both fixed, the magnitude and direction of the final displacement vector will be the same regardless of the actual path followed.
Motion in a straight line
The simplest case for which you will be required to find the speed, velocity or displacement of something is for a point object moving in a straight line, i.e. within a one-dimensional frame of reference. The start and end points for the movement, which we will label x0 and x1 respectively, are specified with reference to their positions relative to an arbitrarily defined point of origin, which we will label O. The diagram below illustrates such a movement.

The point object moves from x0 to x1
The displacement is defined as follows:
Δx ≡ x1 - x0
The upper-case Greek letter Delta is the difference operator, and is used here to denote the change in the value of x. The operator that looks a bit like an equals sign with three horizontal bars is used to denote equality by definition. In other words, we are saying that the change in x is, by definition, equal to x1 minus x0.
In order to be able to determine the velocity of our point object, we need to be able to determine not only its displacement, but the time interval over which the displacement occurs. This will be the difference between the time at which the movement started, and the time at which it ended, as shown below.
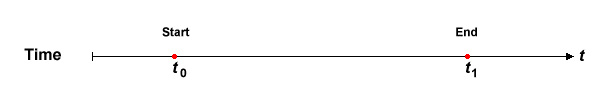
The movement begins at time t0 and ends at time t1
The time interval is a scalar value, and is defined as follows:
Δt ≡ t1 - t0 > 0
Note that the time interval must be greater than zero, unlike displacement which can have a negative value if the movement of the point object is in the negative direction (in diagrams illustrating one dimensional motion, the generally accepted convention is that the point object is moving in the positive direction when it moves from left to right, and in the negative direction when it moves from right to left).
The study of a one-dimensional movement may appear to be a rather simplistic approach to learning about motion, even though there are some real-world examples of one-dimensional motion - a car moving along a straight stretch of road or a sprinter in a hundred-metre race, for example - but many problems that involve two- or three-dimensional motion can be solved by breaking them down into two or three one-dimensional components.
Average vs. instantaneous values
Hopefully you can now see that speed and velocity, although obviously closely related concepts, are not the same thing. One of the most important differences is that, whereas the speed of an object that is moving must have a positive value, its velocity may be either positive or negative, depending on the direction in which it is moving within some defined frame of reference.
We can walk backwards and forwards between two points all day long or run around a field as many times as we like, but every time we return to the point at which we started, we will have a net velocity of zero, no matter how fast we move. If our total displacement (i.e. change in position) amounts to zero, so will our average velocity. We can express the concept of average velocity as follows:
| average velocity = | displacement |
| time elapsed |
Let's look at a graph of (one-dimensional) displacement versus time:
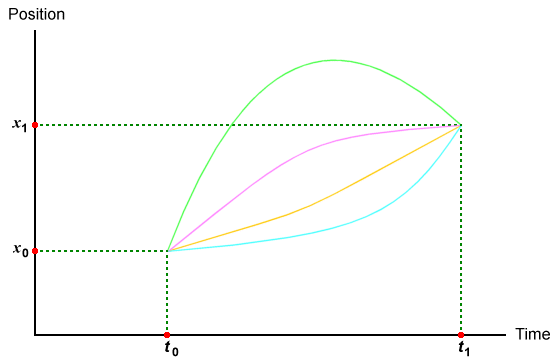
Each path, from start point to end point, has the same average velocity
The graph illustrates the fact that although the distance travelled by an object between the start and end points of a displacement can vary in length, if the time interval in each case is the same, then the average velocity for each path will also be the same. The formula for average velocity is essentially the same as formula we saw when we looked at velocity earlier. We can express average velocity mathematically as follows:
| v ≡ <v> ≡ | Δs |
| Δt |
Note that placing a bar over a variable name or enclosing it between angle brackets indicates that this is the average (or mean) value of the variable. It might occur to you that finding the average velocity is of limited value in terms of what it tells us. As we have seen, an object can move at great speed over a considerable distance, but if it returns to its starting point it will have a velocity of zero. What is often of far greater interest is an object's instantaneous velocity.
In order to determine instantaneous velocity at any given moment, we must find the average velocity over an infinitesimally small interval of time. In other words, we are finding the limit of the average velocity as the length of the time interval approaches zero. This gives us the instantaneous rate of change of displacement. We can express this mathematically as follows:
| v(t) = | lim | Δs | ≡ | ds | ≡ ṡ |
| Δt→0 | Δt | dt |
Note the dot over the variable s on the far right-hand side of the equation, sometimes called an overdot. An overdot above a symbol is commonly used in mathematics to indicate a derivative taken with respect to time. This was also the notation used by Newton for derivatives (or fluxions, as he called them).
Now let's consider speed. We stated earlier that speed is the total distance travelled divided by the total time taken to travel that distance. We should perhaps clarify at this point that what that calculation actually gives us is the average speed for the total distance travelled:
| average speed = | total distance |
| time elapsed |
Distance is a scalar value that always either increases or stays that same during a time interval, whereas displacement can increase, decrease or stay the same. Consequently, the total displacement will always be less than or equal to the total distance, and the average speed will therefore always be greater than or equal to the average velocity.
There are one or two things that the average speed doesn't tell us - like how fast an object is moving at any given moment, or whether the object was stationary at any point. Nevertheless, average speed tells us a lot more than average velocity. For example, if we know the average speed for a journey and the total time taken to complete it, we can work out the total distance covered. Alternatively, if we know the total distance covered and our average speed, we can calculate the duration of our journey. And so on.
We can express average speed mathematically as follows:
| v ≡ <v> ≡ | Δs |
| Δt |
Even though knowing the average speed of an object is often useful, real-world objects rarely move at the same speed all the time, or even for most of the time. Whilst driving a car, for example, we will travel at different speeds, depending on road conditions, traffic, and (of course!) any speed limits in force. We will often want to know our speed at a given moment in time (i.e. our instantaneous speed) to ensure that we don't exceed the speed limit.
We said earlier that speed is a component of velocity (the other component being direction). In fact, the instantaneous speed of an object is the magnitude of the instantaneous velocity. The only difference is that, whereas the instantaneous velocity of an object has a direction that is tangential to the path of the object, the instantaneous speed does not. We can therefore express instantaneous speed mathematically as:
| v(t) = |v(t)| = | lim | Δs | ≡ | ds | ≡ ṡ |
| Δt→0 | Δt | dt |
Tangential and rotational speed
We know that speed is the distance travelled per unit time. Tangential speed is a special case in which we consider the speed of an object moving along a circular path. Imagine a point on the outer edge of a spinning disk. The distance travelled by the point during one complete rotation of the disk will depend upon the diameter of the disk, and the rate at which the disk is spinning (i.e. its rotational speed, which we will return to in due course).
A point closer to the centre of the disk will also make one complete rotation during the same time interval, but its circular path around the centre of the disk will have a much smaller diameter than the point on the outer edge of the disk. Its tangential speed is therefore significantly slower than that of the point on the outer edge. The diagram below shows two points on a spinning disk. Point P1, on the edge of the disk, will have a greater tangential speed than point P2, which is closer to the centre.
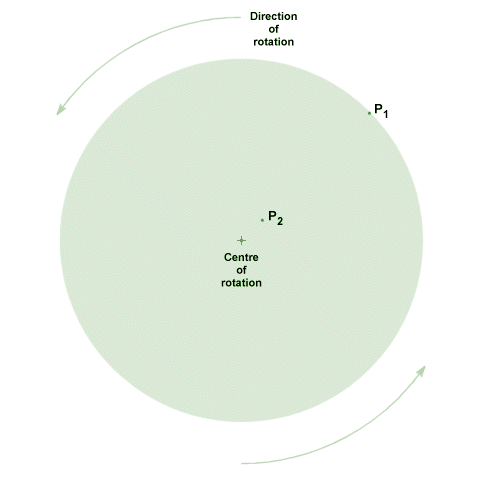
Point P1 has a greater tangential speed than point P2
The speed of an object along a circular path is called its tangential speed because its direction of motion at any given moment is tangent to the circular path. The tangential speed of a point on a rotating disk is dependent upon the rotational speed (ω) of the disk and the radial distance (r) of the point from the centre of rotation. We will look at a formula for tangential speed shortly.
Rotational speed is a measure of the number of complete revolutions that occur per unit time. We often talk about the rotational speed of things in terms of revolutions per minute (rpm). For example, the disks in the hard drive in your computer will typically be spinning at a speed of 5,400 or 7,200 revolutions per minute.
When physicists talk about the rate at which something is rotating, they prefer to use the term angular velocity, which is defined as the rate of change of angular displacement relative to the origin per unit time. Both angular velocity and rotational speed are (usually) represented using the lower case Greek letter omega (ω). The SI unit of angular velocity is radians per second (rad/s). Rotational (angular) velocity is a vector quantity for which the magnitude is the rotational speed.
Every point on the surface of a spinning disk will make one complete rotation in the same amount of time, regardless of its distance from the centre of the disk. Thus, even though a point on the outer edge of the disk will have a greater tangential speed than a point near the centre of the disk, they will both have exactly the same rotational speed. Tangential speed v and rotational speed ω are related by the following formula:
v = rω
Consider a disk five metres in diameter that completes one full rotation every three seconds. What is the tangential speed of a point at the edge of the disk? Since there are 2π radians in one complete rotation:
| ω = | 2π |
| 3 |
And, since our disk has a radius of five metres, we can calculate the tangential speed (in metres per second) as follows:
| v = rω = 5 × | 2π | = 10.472 m/s |
| 3 |
As you can no doubt see, the tangential speed of any point at a fixed distance from the centre of a rigid rotating disk is directly proportional to the rotational speed of the disk. The tangential speed of a point at the centre of a rotating disk will always be zero. If the disk has a fixed rotational speed, the tangential speed of any other point on the disk will be directly proportional to its distance from the centre of the disk:
v ∝ rω
For a point at a fixed distance from the centre of a rotating disk, doubling the rotational speed of the disk will double the point's tangential speed; if on the other hand the rotational speed of the disk remains constant, then doubling the distance of a point from the centre of the disk will double its tangential speed.
Relative speed and velocity
Centuries ago, long before the German theoretical physicist Albert Einstein (1879-1955) formulated his special and general theories of relativity, scientists and philosophers like Galileo and Newton were of the opinion that space and time were absolutes. They nevertheless acknowledged that speed and velocity can only be measured within some frame of reference - a concept broadly known as "relativity".
Before Einstein turned physics on its head in the early twentieth century, it was thought that the time interval observed to elapse between any two events would be the same for any observer, regardless of the distance between the observers or the speed and direction in which each observer was moving with respect to the other. This Galilean relativity (sometimes called Newtonian relativity) relies on the concepts of absolute time and a fixed three-dimensional (Euclidean) geometry for the structure of space.
Newton himself recognised that the speed of a moving object could only be defined with reference to some other object, and that this second object would in all probability itself be in motion. The planets in the solar system, after all, are constantly in motion with respect to the Sun and each other; the Sun itself is in the outer reaches of a spiral galaxy that is spinning around its centre, and the galaxies themselves are moving relative to each other.
Nevertheless, Newton believed that, somewhere in the universe, there must be some fixed point that was completely at rest. He believed that - in theory at least - true speed measurements could be made with reference to that point. We know now that this is not the case, and that the relationship between space and time is rather more complex than Galileo and Newton imagined.
Let's start by thinking about what we actually mean by "relative velocity" in a one-dimensional (and non-relativistic) sense. Imagine an observer standing on the platform at a railway station as a passenger train passes him or her at a constant speed of twenty metres per second (20 m/s). A man is walking along the top of the train in the opposite direction at a constant speed of one-and-a-half metres per second (1.5 m/s) relative to the train.
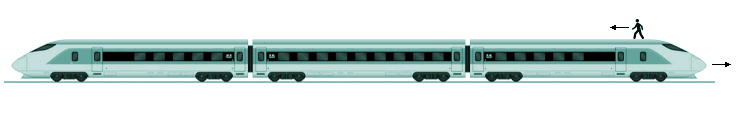
A man walks along the top of a train in the opposite direction to that in which the train is moving
If we consider the platform (and hence the observer) to be at rest, then the velocity of the man relative to the observer will be the sum of the velocities of the train and the man. We can express this mathematically as follows:
vMO = vTO + vMT
where:
vMO is the velocity of the man M relative to the observer O
vTO is the velocity of the train T relative to the observer O
vMT is the velocity of the man M relative to the train T
You may see different notational conventions used to denote that a value is being specified relative to particular reference point - for example, vMO could be written as vM|O or vM rel O. In any case, if we substitute actual values into our equation we get:
vMO = vTO + vMT = 20 m/s + (-1.5 m/s) = 18.5 m/s
Thus, the relative velocity of the man, in the rest frame of the observer, is 18.5 metres per second (18.5 m/s) in the direction in which the train is moving. All we have done is add the two velocities together. From a relativistic point of view (as we shall see), the answer we have arrived at is not strictly correct. But, until we start thinking in terms of velocities that have magnitudes approaching a significant fraction of the speed of light, it gives us a good enough approximation for most practical purposes.
Inertial frames
Imagine yourself inside a capsule in space with no windows. Assume that the capsule is moving in a straight line at constant speed. How would you determine your speed and direction of travel? Because the capsule is not undergoing acceleration, you would have no sensation of movement. In fact, there would be no experiment you could carry out to determine your speed or direction of movement - or even to determine whether you were moving at all.
We said above that speed and velocity are relative terms. The velocity of an object can only be determined relative to some other object or set of coordinates in space and time. Unfortunately - and contrary to what Newton imagined - there is no fixed point anywhere in the universe that is completely at rest. We can nevertheless define an inertial frame of reference from which to measure the velocity of an object that is of interest to us. But what exactly do we mean by inertial frame of reference?
Newton's first law of motion (the law of inertia) states that if the net force (i.e. the vector sum of all of the forces acting on an object) is zero, then the velocity of the object is constant - which is another way of saying that it is either at rest or moves in a straight line at a constant speed (we will be looking at Newton's laws in more detail elsewhere in this section).
We can define an inertial frame of reference as one in which a body has zero net force acting upon it and is not accelerating. It will appear to be either at rest or moving at a constant velocity (i.e. moving in a straight line at constant speed) when viewed from that frame. An inertial frame is thus one in which Newton's first law of motion is obeyed.
Think about the example of the moving train described earlier, and consider the observer standing on the platform in the railway station. As far as the observer is concerned, he or she is at rest, i.e. not moving. Both the train and the man walking along the top of the train (who is doing so for reasons we can only guess at!) are moving at constant velocities relative to the observer. The observer measures both the velocity of the train and the velocity of the man relative to his or her own frame of reference.
In the train scenario, we can identify three different viewpoints - that of the observer standing on the platform, that of the man walking along the top of the train, and that of an imaginary passenger sitting on the train. Each of these viewpoints can be considered to be an inertial frame, and each of these inertial frames is in constant rectilinear motion (i.e. motion in a straight line) with respect to the others.
Newton's law of inertia will work equally well for the observer standing on the platform and for a passenger on the moving train. Experiments carried out by the observer on the platform and the passenger in the train will produce exactly the same results. Both the observer and the passenger, for example, could juggle a set of balls in exactly the same way; the physics is the same in each case.
In the strictest sense, an inertial frame is defined as one that is free of external forces. In practice, however, this is virtually impossible to achieve. The inertial frames in our train scenario, for example, are all subject to acceleration due to the Earth's gravitational field. Nevertheless, two inertial frames that move with a constant velocity with respect to one another when not being accelerated will maintain the same behaviour when both are subject to the same acceleration - i.e. the acceleration must be of equal magnitude and acting in the same direction.
With this proviso in mind, we can broadly define a collection of inertial frames as a set of frames that are either stationary or moving at a constant velocity with respect to one another, and for which all of the frames in the set, together with everything observed within each frame, are subject to a common acceleration.
An introduction to special relativity
Albert Einstein published his special theory of relativity in 1905. The theory challenged the conventional views of the day concerning the relationship between space and time. Instead of the Galilean relativity, in which time is absolute and the structure of space has a fixed three three-dimensional geometry, Einstein described a four-dimensional space-time continuum.
In this space-time continuum, the three spatial dimensions (left-right, up-down, and backwards-forwards) are inextricably linked with time, which becomes the fourth dimension. Space and time are no longer regarded as separate physical constructs. Physicists could now describe any event or physical phenomenon in terms of its location within the space-time continuum.
Note that we are only concerned here with inertial frames of reference. Special relativity can also be applied to non-inertial frames of reference, but they are handled somewhat differently. Note also that, when Einstein first proposed his theory of special relativity, he did so on the basis of a flat space-time continuum that did not take into account the effects of gravitational forces.
Essentially, if these forces are negligible, special relativity gives a good enough approximation in most cases (in the same way that Newtonian mechanics gives a good enough approximation at speeds that are not a significant fraction of the speed of light). Once we start factoring in significant gravitational forces, we need to think in terms of what Einstein described as curved space-time. Einstein's general theory of relativity basically extends special relativity to take into account the distortion of space-time in the vicinity of a massive object due to that object's gravitational field.
Special relativity makes two fundamental assumptions. The first is that the laws of physics are exactly the same in two different inertial frames of reference - a concept it has in common with Galilean relativity. The second is that the speed of light (symbol: c) is the same for all observers regardless of the motion of the observer and regardless of the motion of the light source itself.
In order to understand why this second assumption is so important, we need to consider the work of the Scottish mathematician and physicist James Clerk Maxwell (1831-1879) whose work on electromagnetic radiation led him to the discovery that light was part of the electromagnetic spectrum, and as such was able to propagate through space in the form of an electromagnetic wave. Maxwell assumed that, in order for this to be possible, some unseen medium (which he called "aether" after the Greek god of light) must permeate the fabric of space.
The assumption is not unreasonable, given that other kinds of waves require a medium in which to propagate. Water waves, for example, cannot exist without water, and sound waves cannot propagate in a vacuum. The absence of any tangible evidence for the existence of such a medium led the American physicists Albert Abraham Michelson (1852-1931) and Edward Williams Morley (1838-1923) to carry out their now-famous experiment of 1887, which was designed to prove that "aether" really did exist.
Michelson and Morley reasoned that if aether existed, then the Earth (which orbits the sun at a velocity in excess of 100,000 kilometres per hour) would create an "aether wind" as it moved through the aether. They used a device called an interferometer in an attempt to measure the speed and direction of this aether wind by measuring the speed of light moving in different directions.
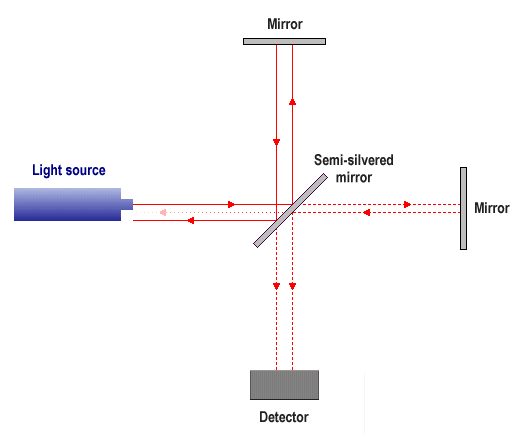
Schematic of an interferometer of the kind used in the Michelson-Morley experiments
The device generates a beam of light which is directed towards a semi-silvered mirror angled at forty-five degrees to the light source. The semi-silvered mirror acts as a "beam-splitter", allowing some of the light to pass straight through it in the direction of a standard mirror located opposite the light source. The remaining light is reflected through an angle of ninety degrees towards a second mirror located directly above the beam splitter.
The light arriving at the two standard mirrors is reflected back towards the beam splitter. Some of the light from each reflected beam is directed downwards by the beam splitter towards a detector. Michelson and Morley had expected to see interference patterns at the detector that would indicate differences in the speed of the two beams due to the effects of the "aether wind", but they were unable to detect any discernible difference.
Einstein believed that this failure to detect "aether" was because no such medium existed; that light did not in fact require a medium in which to propagate, and that the speed of light was thus the same for all observers regardless of how fast, or in which direction, they were moving. This belief was consistent with the results of various experiments, and with the mathematics underpinning the laws of physics. It did however have some rather strange consequences.
According to Einstein's special theory of relativity, nothing in the universe - matter, energy or information - can move faster than the speed of light. The speed of light c has been determined experimentally to be slightly less than three hundred thousand kilometres per second (299 792 458 m/s). And, because this "cosmic speed limit" is the same for any observer, two observers in different inertial frames will perceive time differently.
Imagine that you are on board a space ship travelling at a constant velocity of half the speed of light (0.5 c). Because your velocity is constant, the spaceship is an inertial frame of reference, and the laws of physics will be exactly the same for you as they would for an observer in any other inertial frame of reference. In fact, because your velocity is constant, you will have no sensation of movement.
Now suppose you hold a flashlight at a height of exactly one meter above the floor of the space ship and point it straight up at a ceiling mirror two metres above the floor of the spaceship. A beam of light from the flashlight strikes the mirror and is reflected downwards towards a light detector on the floor of the spaceship, as shown in the illustration below. How far has the light travelled when it enters the detector?
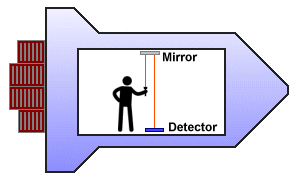
A beam of light directed towards a ceiling mirror is reflected towards a detector on the floor
You might be tempted to say that the light has travelled a total distance of three metres (one metre from the flashlight to the ceiling mirror, and two metres from the ceiling mirror to the detector on the floor). And from your point of view, i.e. in your inertial frame of reference, you would be correct. But suppose that, at the precise moment you turned on the flashlight, you were whizzing past a stationary observer in space? You are travelling at half the speed of light, so what would the observer see?
Consider the fact that the speed of light, although very, very fast, is finite. Given the speed at which you are travelling, your spaceship is going to be in a different place when the light from your flashlight hits the detector from where it was when the light actually left the flashlight. You, in your inertial frame, will see the beam of light going straight up and down. A stationary observer will see something rather different, as illustrated below.
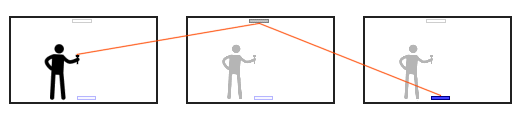
A stationary observer sees the beam of light follow a diagonal path
We have stated already that the speed of light is the same for all observers (we say that it is invariant). That being so, then what are the implications of a stationary observer seeing the light travelling over a greater distance than you do in your space ship frame of reference? Clearly, the time interval between the light leaving the flashlight and its arrival at the detector will be greater for the observer than it is for you.
From your point of view, standing on your spaceship, nothing has changed. You do not experience anything out of the ordinary. From the observer's point of view, however, time appears to pass more slowly on the space ship than it does in their own frame of reference - a phenomenon known as time dilation. It follows that, from the observer's point of view, you will be moving somewhat slower than 0.5 c.
In fact, given two different observers in two different inertial frames moving with a constant velocity, each observer will see time passing more slowly in the other's frame of reference - a phenomenon known as mutual time dilation. This has a number of interesting consequences - especially when we start thinking about things like relative speed.
We don’t want to delve too deeply into relativity just yet, but a relatively simple example should serve to illustrate the kind of thing we are talking about. One question that seems to come up time after time when students start learning about relativity goes something like this:
"OK, I understand that nothing can travel faster than light, but suppose two space ships are travelling directly towards each other at nearly the speed of light. Surely, the speed of one space ship relative to the other must exceed the speed of light?"
Let's assume, for argument’s sake, that it will one day be possible to build spacecraft with near light speed capabilities. Suppose we have two space ships as shown in the diagram below heading directly towards each other, each moving at a significant fraction of the speed of light. Space ship A is moving with a velocity of vA, and space ship B is moving with a velocity of vB.
Space ships A and B are headed directly towards each other
At relatively low speeds, we could simply express the combined approach speed vAPPR as the sum of the magnitudes of the two velocities vA and vB - which would give us a good enough approximation - as follows:
vAPPR = |vA| + |vB|
At speeds that are a significant fraction of the speed of light, however, the relativistic effects will become too great to ignore. From an external frame of reference, we may observe A and B approaching each other, each moving at a significant fraction of the speed of light. But how will things look to an observer on either of these space ships? To find the velocity of B relative to A, we use the following formula:
| vBA = | vB - vA | |
| 1 - | vB·vA | |
| c2 | ||
Where c is the speed of light (299 792 458 m/s). Note that, for this one-dimensional example, we will adopt the convention that velocities moving from left to right are positive. Because B is moving from right to left, its velocity will be negative. Let's put some actual figures into the equation and see what result we get. We will assume that (from our external frame of reference) A and B are moving at 0.65 c and -0.85 c respectively:
| vBA = | -0.85 c - 0.65 c | |
| 1 - | -0.85 c · 0.65 c | |
| c2 | ||
| vBA = | -1.5 c |
| 1 - (-0.5525) |
| vBA = | -1.5 c |
| 1.5525 |
| vBA = -0.966 c |
You should be able to see from this somewhat simplistic example that, regardless of how close to the speed of light our two spaceships manage to travel, their speed relative to one another will never exceed the speed of light. We will be looking at both special and general relativity elsewhere, but at least you now have some idea of some the strange things we can expect to see when we start dealing with relativistic velocities!
